9 Teaching Quantum Physics in Cambridge: George Birtwistle and His Two Textbooks

Shortly after the end of the Great War, Charles Galton Darwin, a former student of Trinity College, Cambridge, and later fellow and lecturer at Christ’s College, wrote a letter to his friend Niels Bohr complaining about the situation of the quantum theory in the old university. From his point of view:

[P]hysics and applied mathematics here are in an awful state. I am doing my inadequate best to talk to people about quanta; everybody accepts them here now (which is better than it was in 1914 at any rate), but I don’t think most of them realize their fundamental importance or have studied the arguments in connection with them […]. There are plenty of very intelligent people, only under the blighting influence of studying such things as strains in the ether, they none of them know what it is worth doing.¹

By 1927 things had changed. The “Mathematical Tripos” (MT) and the “Natural Science Tripos” (NST) not only included a number of courses on quantum matters, but students taking these subjects were expected to respond to questions that, only some months earlier, had troubled the best scientific minds. To give an example, in the spring of 1928, one of the questions in the final exams was the following: “Show how the Heisenberg matrix of a 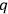-number is determined from the normalized Schrödinger characteristic functions (Eigenfunktionen) of the problem concerned. Illustrate it by the problem of the rigid rotator (molecule).”² This question expected an understanding not only of Werner Heisenberg’s and Erwin Schrödinger’s theories of quantum mechanics, but also their equivalence, all of which had been developed only two years earlier. Some students in Cambridge were thus, at this stage, quite up-to-date with contemporary quantum questions, enabling them to become actors tout court in the developments of the new physics.

How did this change come about? The development of quantum physics and early quantum mechanics is a story that skips Cambridge and, generally, the British world. The first main English actor, Paul Adrien Maurice Dirac, appears on stage only in the second half of the 1920s. In the background, people like James H. Jeans, Ralph H. Fowler, and Charles G. Darwin play merely secondary roles in the grand narrative of quantum physics. However, these and other characters are instrumental to the understanding of how the theory arrived and took root in Cambridge.

Here, I contribute to the early history of quantum physics in Cambridge by directing attention to the pedagogical side of the story. In particular, I concentrate on two books written by a quite-unknown Cambridge don, George Birtwistle (1877–1929). A senior wrangler in 1899, Birtwistle was fellow and lecturer of mathematics at Pembroke College and lectured on quantum physics and quantum mechanics between 1924 and 1929, producing two books that comprise his lectures. These two books present a number of interesting aspects. First, they help us understand the way a generation trained in the old wrangler tradition could understand and teach quantum theory. Second, they characterize the content that non-specialists in Cambridge received about the new physics. And third, they embody the tensions experienced by lecturers and students of the quantum theory at a time when it was developing and transforming rapidly.³

In the first section (9.1), I review British scientists’ early responses to quantum physics. The 1913 meeting in Birmingham of the British Association for the Advancement of Science (BAAS) was the first major public event in Britain in which positions in favor and against the theory of the quanta were discussed at length. Jeans, one of the first British converts to Planck’s theory, wrote a report on the status quo of the quantum. This short book eventually became the source from which many British physicists got their first knowledge about the theory of quanta, during and immediately after the war. In section (9.2), I explain the evolution of teaching quantum theory in Cambridge, looking at the list of courses, examinations, and lecturers. This leads us to the two books by Birtwistle, The Quantum Theory of the Atom (1926) and The New Quantum Mechanics (1928a), in sections (9.3) and (9.4), respectively. These two books may be channels for understanding the situation of the quantum in the Cambridge lecture room: undergraduate students had up-to-date resources, locally produced, through which they could keep up with the latest developments in quantum physics and quantum mechanics. As we shall see, some of these resources were not necessarily the best tools to grasp the radical novelty of the new theories.

9.1 James Jeans and His Report on Radiation and the Quantum-Theory

The first written reference to Planck’s hypothesis in the British scientific milieu was probably Joseph Larmor’s explicit rejection of it at the 1902 BAAS meeting. In the following years, the general attitude in Britain ranged from total opposition to oblivion but was, generally, one of skepticism. Ten years later, however, and after the first Solvay Conference in 1911, the increasing presence of Planck’s hypothesis in the scientific literature forced a new discussion of the topic in the same forum: the BAAS meeting in Birmingham in the summer of 1913. Jeans, who had recently converted to the theory of the quantum and was one of only two British physicists present at the Solvay meeting, took on the task of explaining and defending the theory of the quanta to a reluctant audience.

Jeans had been second wrangler in 1898, being one of the first two students, together with Godfrey H. Hardy, to attempt the Cambridge Mathematical Tripos in only two years—and not in the usual three years—after which he was appointed fellow and lecturer in Trinity College (Milne 1952). During this period, he worked on radiation theory and statistical mechanics, producing his first book, The Dynamical Theory of Gases (Jeans 1904), and contributing to what we now know as the Rayleigh-Jeans law for the distribution of the radiation from a black-body, which was derived using the equipartition of energy. His constant failure to describe the experimental energy distribution of black-body radiation using classical arguments did not force Jeans, at first, to accept Planck’s hypothesis, but to search for alternative mechanisms to explain the experimental law. Faithful to the equipartition principle, a central tenet in statistical mechanics, Jeans was first willing to challenge Planck’s law on the basis that real, thermal equilibrium was impossible in a black body. But by 1910 he had changed his mind, forced by the explanatory success of Planck’s law as well as by the theoretical proof that this law could be obtained only with the assumption of quanta (Hudson 1989). Another recent convert, Henri Poincaré, also developed a very detailed demonstration of the sufficiency and necessity of the hypothesis of quanta for obtaining Planck’s law in 1912, just after the first Solvay conference. Jeans admired Poincaré’s more general proof, and he used it in his subsequent defense of the quantum theory.

The Report on Radiation and the Quantum-Theory that Jeans prepared for the 1913 BAAS meeting, and which was published a few months later, acted as a textbook from which many British scientists learned the basic tenets of the quantum theory during the war, or immediately afterwards (McCrea 1985). That is why it serves as the starting point for this pedagogical story, even though it was not formally a textbook. The Report also offers a window into Jeans’s own conversion process, emphasizing the impossibility of accounting for black-body radiation with any hypothesis other than Planck’s quanta and, also, stressing the importance of Poincaré’s reflections and Bohr’s model of the atom. Albert Einstein’s explanation of the photoelectric effect, and the theory of the specific heats of solids by Einstein, Peter Debye, and Frederick A. Lindemann are also present, but only as indirect support for the quantum hypothesis.

The Report is an interesting exercise of rhetoric, intended to convince British mathematical physicists, mostly influenced by the MT Cambridge tradition, of the unavoidability of the quantum hypothesis. From the beginning of the book, Jeans addresses the same criticisms of Planck’s theory that he himself had offered a few years before, by acknowledging that:

[T]he mere discovery that a phenomenon is difficult to explain in the Newtonian way is no adequate reason for abandoning a system of laws which is known to hold throughout vast regions of natural phenomena […]. From demonstrating that a matter is difficult to proving that it is impossible is a long step, but if this step can be taken with respect to the explanation of even one well-established phenomenon of Nature, then the logical necessity of rejecting the impossibility becomes unanswerable. (Jeans 1914, 2)

The tendency in Britain at the time was to follow in Larmor’s footsteps, who was still trying to obtain Planck’s law in terms of some continuous motion or mechanism, in spite of Jeans’s and Poincaré’s demonstration of the fundamental impossibility of such a project (see for example Larmor 1909; Hudson 1989, 72). For instance, Augustus E. H. Love, second wrangler in 1885 and Sedleian Professor of Natural Philosophy in Oxford since 1898, argued that “from a mathematical point of view there must be infinitely many formulae which would agree equally well with the experiments” (Anonymous 1914, 384, see also Ewald 1913). Larmor himself, and Joseph John Thomson, were the main opponents to Jeans, this time also rejecting the new theory of specific heat in solids, while Hendrik Antoon Lorentz and a young Bohr were on Jeans’s side. The discussions at the Birmingham meeting of the BAAS “made it abundantly clear that the quantum theory is far from being regarded as inevitable yet by many of the English school of physicists” (Jeans 1914, 23), and that is why Jeans took in the Report a very pedagogical approach, including full references to the criticisms by Love, Thomson, Larmor and others, and his answers to those challenges. Incidentally, the BAAS meeting started with a presidential address given by Oliver Lodge on “Continuity,” a manifesto in favor of the real existence of the ether, its essentially continuous nature, and against the theories of relativity and quanta (Lodge 1914).

To understand the Report, we have to bear in mind the mental framework of the public to which it was addressed, a framework which Jeans himself had, until very recently, fully shared, and which had its roots in the metaphysics embedded in the training of Cambridge mathematical physicists. The ether was a real substance—and this remains so in the Report—and physical explanation was synonymous with mechanical modeling. These two aspects were pivotal in the introductory chapter:

For whatever is regarded as certain or uncertain about the ether, it must be granted as quite certain that it approaches more closely to a continuous medium than to a gas […]. And if, as seems most probable, the ether is a perfectly grainless structure, […] the total energy [in a black-body] will be infinite. […] To put the matter shortly: in all known media there is a tendency for the energy of any systems moving in the medium to be transferred to the medium and ultimately to be found, when a steady state has been reached, in the shortest vibrations of which the medium is capable. This tendency can be shown (Chapter II) to be a direct consequence of the Newtonian laws. This tendency is not observed in the crucial phenomenon of radiation; the inference is that the radiation phenomenon is determined by laws other than the Newtonian laws. (Jeans 1914, 6–7)

In support of the latter, chapter 2 partly repeats Jeans’s own work from before 1910, in which he tried to exhaust all possible mechanisms that might account for the “full radiation” or “black-body radiation” with classical arguments. The core of the argument was, obviously, that “any radiation formula corresponding to a steady state must be derived by expressing that the amount of energy gained by the ether is equal to the amount absorbed” (Jeans 1914, 9), for which one had to think of different possible mechanisms of absorption and emission. Jeans tested three such possibilities: “resonators” of perfectly definite periods, the motion of free electrons in matter, and the photoelectric effect. In all cases, he obtained the Rayleigh-Jeans formula he had obtained from the general principle of equipartition, and therefore, he inferred that the ultraviolet catastrophe was unavoidable on classical grounds: “It is to escape from this necessary consequence of the classical mechanics that the quantum theory has been brought into being” (Jeans 1914, 23).

Chapters 3 to 6 give a very clear account of the quantum theory and its success in accounting for radiation, spectra, the photoelectric effect, and the specific heat of solids (in this order), leaving for the last chapter what he calls the “physical difficulties” or the “physical basis” of the theory (Jeans 1914, 33 and 79). And this is the chapter to which I now turn, because it is here that we find Jeans trying to understand, or better to speculate on, the physical implications of accepting the quantum theory. Because, as he well says, accepting Planck’s hypothesis tells us very little about the reality of physical processes:

The indications are that there is, underlying the most minute processes of nature, a system of mechanical laws different from the classical laws, expressible by equations in which probably the quantum-constant h plays a prominent part. But these general equations remain unknown, and at most all that has been discovered is the main outline of the nature of these equations when applied to isochronous vibrations. (Jeans 1914, 79)

The main problem for Jeans was not that the quantum theory was, as yet, limited in its applicability, but that “even if the complete set of equations were known, it might be no easy task to give a physical interpretation of them, or to imagine the mechanism from which they originate” (Jeans 1914, 79, emphasis added). I emphasize the last sentence because, for him, as for most physicists of the Cambridge school, intelligibility involved the possibility of imagining a mechanism that could account for the observed phenomena. But when faced with the quantum, any “attempt to imagine a universe in which action is atomic leads the mind into a state of hopeless confusion” (Jeans 1914, 79–80).

From dimensional considerations, Jeans underlined that Planck’s constant had the physical dimensions of angular momentum, something consistent with Bohr’s recent theory for the hydrogen atom. In any case:

[T]he brilliant agreement […] with experiment may indicate that in these cases the angular momentum of the single electron certainly behaves as though it were atomic, but this does not carry us any perceptible distance towards a physical explanation of why this atomicity exists. (Jeans 1914, 80)

More interesting for Jeans, and also from dimensional considerations, h is related to the square of electric charge, which meant it was related to “the strength of a tube of force binding two electrons. This suggests that the atomicity of h may be associated with the atomicity of e” (Jeans 1914, 81). Jeans reminded the reader that the atomicity of the electrical charge had no basis in Maxwell’s theory, and that, so far, “no reason is known why an electron with charge e should not exist” (Jeans 1914, 81). And, although the atomicity of the charge did not necessarily involve the quantum theory, “otherwise the quantum theory would have been fully developed long ago […] there is, perhaps, a hope that the two atomicities may be special aspects of some principle more general than either of them” (Jeans 1914, 81); and this had to be, inevitably, related to the structure of the ether.

The incorporation of Thomson’s “tubes of force,” a very Cambridge mathematico-mechanical device, is, I think, suggestive of the fact that Jeans was not willing to do away with the Cambridge tradition to which he belonged. Jeans regarded Einstein’s hypothesis of a quantum as “corpuscles of radiation” comparable to Thomson’s real existence of discrete Faraday tubes. Both constructions could account for the structure of energy exchanges, only that the latter would be in continuity with the older framework. But in both cases there was no hope of reconciling the undulatory theory of light with the quantum theory, since experimental evidence “seems almost to indicate that both theories are true simultaneously” (Jeans 1914, 89).

This last chapter finishes with a discussion on the reality of the ether, acknowledging that, in this respect, continental and British physicists play on different—opposed—sides. Jeans seems to cling to the reality of the ether, but he relegates it to a second place: the real stumbling block being the contradiction between discrete and continuous theories, both valid for different radiation phenomena. And, with this, the last pages of the book convey a certain amount of pessimism as to the status quo of physics. In a free translation from Poincaré’s Dernières Pensées he says:

It is impossible at present to predict the final issue. Will some entirely different solution be found? Or will the advocates of the new theory succeed in removing the obstacles which prevent us accepting it without reserve? Is discontinuity destined to reign over the physical universe, and will its triumph be final? Or will it finally be recognized that this discontinuity is only apparent, and a disguise for a series of continuous processes? […] Any attempt at present to give a judgement on these questions would be a waste of paper and ink. (Jeans 1914, 90)

While chapters 2 to 6 were an active exercise in convincing the reader of the inevitability of the quantum hypothesis and its successes, these last pages blunt that optimism by pointing to the difficulties of interpretation of the quantum theory. But this is done in a particular way: these last sentences can be interpreted as a way to encourage British physicists to embrace the theory rather than a priori rejecting it on the grounds that it is not “physical,” that is, mechanical. Furthermore, the fact that these considerations appear only at the end of the book as a separate chapter may indicate that, from Jeans’s point of view, one could and should accept the quantum theory without having a full answer to its ultimate physical meaning. Partly following the problem-solving tradition of the Cambridge MT pedagogy, Jeans was more concerned about proving that the quantum theory solved specific problems than attempting an overall challenge on metaphysical grounds.

9.2 Teaching Quantum Theory in the 1920s

As mentioned in the introduction, the position of quantum theory in Cambridge was far from satisfactory at the end of the Great War. In 1920, Jeans himself, when adding a last chapter on quantum theory in his third edition of The Dynamical Theory of Gases, regrets the absence of British scientists in the new science. He writes:

This chapter can of necessity provide only a very brief introduction into the mysteries of Quantum Dynamics, but I hope it will be of value in stimulating the interest of English-speaking readers in a branch of science of which the development has so far been left mainly to other nations. (Jeans 1921, preface to the third edition)

One way to track the status and evolution of the quantum in the old university is to have a look, however quick, at the evolution of courses taught to undergraduates. The “advanced,” optional courses were normally a reflection of the particular interests of individual researchers, and could give rise to exam questions only in what was known as Schedule B of the Tripos, Part II.⁴

It should be remembered that, following a tradition going back to the 1860s, physics in the 1920s was taught as part of the “Mathematical Tripos” (as theoretical physics or applied mathematics) and as part of the “Natural Science Tripos” (which was mainly experimental science). This meant that these two worlds were relatively independent of each other: experimental physics being taught at the Cavendish Laboratory, and mathematical physics by college lecturers. However, the special optional courses were, for the most part, open to both kinds of students.

Who could teach quantum theory in Cambridge? Certainly not people like Larmor or Thomson who were strongly opposed to it. Nor could Ernest Rutherford, whose program was basically experimental. It was young people, both trained in the Cambridge Tripos and converted to the new theory, who could teach quantum physics. And these were, at the beginning of the decade, Darwin and Fowler. In a recent paper, I discussed Darwin’s early understanding of quantum physics and the evolution of his ideas throughout the decade (Navarro 2009). After his training in the MT, he moved to Manchester, where he learned experimental techniques related to spectra and radioactivity. There, he also met Bohr in the dramatic years of the development of the atom model using the quantum hypothesis. In 1919, he was appointed fellow of Christ’s College and started giving the first courses on quantum theory and its relation to spectra. It is interesting to note that the first such course was primarily meant for NST students, probably supported by Rutherford.

When Darwin left Cambridge in 1922, Fowler began to teach quantum physics, this time in courses open to both triposes. Unlike Darwin, Fowler was self-trained in the theory of quanta and eventually became the catalyst for work in quantum physics in Cambridge, promoting a new generation of quantum physicists by, for example, translating into English many of the key papers that were appearing in German, as well as by inviting people such as Ralph Kronig or Heisenberg to give lectures in Cambridge. He was also a sort of father figure to people like Douglas Hartree, Llewellyn H. Thomas and, of course, Dirac, all of whom made important contributions to the development of quantum physics in the late 1920s. It is also well known that Fowler became a sort of theorist-in-residence at the Cavendish, as well as Rutherford’s son-in-law (Gavroglu and Simoes 2002).

In the academic year 1924/1925, we see a turning point in the teaching of quantum theory in Cambridge. Fowler had been, for two years, giving the only, one-term course on the “Quantum Theory of Spectra.” But that was not enough now. Quantum physics was progressing, and Cambridge started to teach advanced courses. Not surprisingly, it was the younger generation that could teach the latest developments, since they had been in close contact with Copenhagen and some of the German research centers.⁵ Thus, we find advanced courses taught by Dirac and by Hartree in the second half of the decade; courses that were, especially in Dirac’s case, but also in Fowler’s and Hartree’s, reflections of science in the making.

The following is a list of all these courses taken from the information provided in the Cambridge University Reporter in the period 1919–1929:

Tab. 9.1: List of all courses announced in the Cambridge University Reporter in the period 1919–1929.

The only outsider named in the list of lecturers teaching quantum physics is Birtwistle, to whom the rest of this paper is devoted. And I say outsider not because he came from some other university, but because he was the only “real” wrangler accepting and spreading quantum physics in Cambridge, which makes him a unique example in trying to understand the ways in which the new theory was received in the old Cambridge wrangler tradition.

Birtwistle is a typical product of the MT tradition. Born in 1877, he arrived in Cambridge in 1895 and was bracketed senior wrangler in 1899. This means that he was a contemporary of Jeans, but took the usual three years to sit for the MT examination. After this, he was appointed fellow and lecturer of mathematics in his own college, Pembroke, where he remained until his sudden death in May 1929. Like many dons of the old school, “it was as a teacher rather than as an investigator that Birtwistle was known, and as a teacher that he played a conspicuous part in Cambridge mathematics” (Anonymous 1929, 881). The short description of his teaching style in the obituary note we find in Nature is almost all we have about him:

As a lecturer, Birtwistle was admirably clear and easy to follow. He set, in fact, a standard of exposition which made it very difficult for anyone to attract students to any duplicate course. His books are like his lectures—admirable expositions of those sections of the subject with which he deals, written in lecture-room style. He seldom attempts to go deeply into difficult points or to present the subject as a single logical whole. His aim is the lecturer’s aim—to interest the student in the subject, especially in its more outstanding or exciting parts, and lead him on to other more systematic or abstruse expositions. (Anonymous 1929, 881)

What courses did he normally teach? In the annual lists, we find him consistently teaching the general introductory courses on “Mechanics (Statics and Particle Dynamics; Rigid Dynamics)” and “Electricity,” and he was among the first to take on board courses on thermodynamics when these were introduced in the list of elementary courses in 1924. As for his more specialized courses, between 1920 and 1924, he consistently taught a one-term course on “Hydrodynamics (motion of solids and vortices in a liquid; waves).” In the academic year 1924/1925, he started teaching an “Introduction to Quantum Theory,” while Fowler taught more advanced quantum matters. In the following years, he taught further quantum courses, from which he finally produced two books: The Quantum Theory of the Atom in 1926, and The New Quantum Mechanics in 1928.

9.3 The Quantum Theory of the Atom

The Quantum Theory of the Atom is a window into Birtwistle’s first courses on quantum physics, in the early months of 1925, and in the academic year 1925/1926. It consists of a compilation of lectures from that period, and it was intended as a textbook for a similar course the following year (1926/1927). As is obvious from his correspondence with the publisher, Birtwistle rushed the printing of the book for two reasons: “as you know the subject is changing so rapidly that it would be a good thing to get it out as soon as possible; also so far there is no English book of this kind so far published and I think it will meet a real demand.”⁶ This book does not try to give a full, consistent, and closed picture of quantum physics, but rather to teach the mathematical apparatus needed to apply quantum physics, as known at the time. That means that the book is organized around the quantization strategy and its application to those cases for which it works. For the conceptually-minded reader, however, the book is disappointingly flat. Contrary to what happened with Jeans’s Report, and also compared to other pedagogical works, Birtwistle’s book does not provide many explanations concerning the “physical” meaning of the theory; it basically teaches the mathematical methods for applying quantum physics to different problems and shows their agreement with experimental data.

But before we go into these and other technical elements, there is an aspect of the book, present especially in the more historical first two chapters, of particular interest. Birtwistle links the history of quantum physics to developments by British, especially Cambridge, scientists. The Quantum Theory of the Atom describes precisely that: the quantum theory of the structure of the atom, and this is a story that, according to Birtwistle, has its beginnings in Cambridge: “the modern theory of the structure of the atom is in the first place due to J. J. Thomson” with his discovery of the electron (Birtwistle 1926, 16). In this timeline, Thomson’s key contributions continued with his model of the atom, and also with his study of positive rays, since the latter was the source for Francis Aston’s mass spectrograph and the discovery of isotopes. Birtwistle’s story of the structure of the atom continues with Rutherford “and his school in which the instrument of the -particle was used to disclose the nature of the atom” and to propose an atomic model “which is now generally used in theoretical work” (Birtwistle 1926, 17). This model, for instance, is used to explain the nature of Thomson’s positive rays.

In this historical survey, Bohr’s 1913 contribution to the atomic model comes only after a detailed explanation of the hydrogen spectrum and the need to explain Balmer’s formula. But Bohr’s contribution comes hand in hand with the work of another Cambridge researcher, John W. Nicholson, who was working on stellar spectra and who brought forward, in 1912, an atomic theory in which Planck’s constant was interpreted as determining the angular momentum of permissible orbits of the electrons inside an atom.⁷ Birtwistle rightly distinguishes between Nicholson’s and Bohr’s contributions, the former giving only the condition for the angular momentum of an electronic orbit to be where 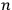is an integer, while the latter gave the “new concept which was to be the key to the solution of the problem of spectra,” namely that “the radiation emitted between transitions between two stationary states has a frequency 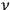given by the relation 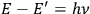” (Birtwistle 1926, 23). Throughout the book, however, Birtwistle keeps the expression “the Nicholson-Bohr condition,” meaning the nuclear model with quantized orbits. For the reader, this British-oriented story consolidates the idea that it was the “amazing verification” of Bohr’s atomic model that “at once fixed attention upon the quantum theory, which up to then had received skeptical regard from physicists in general” (Birtwistle 1926, 24).

The third chapter is a compilation of things that are related to the quantum theory but that are not dealt with in detail in the book. First is the one-page explanation of the mathematics of Bohr’s correspondence principle, in the version he introduced in his 1918 paper “On the Quantum Theory of Line Spectra” (Bohr 1918). After this rather plain introduction of the correspondence principle, chapter 3 continues with a section devoted to the photoelectric effect, and another section in which he explains Einstein’s 1917 deduction of Planck’s radiation formula. On the former, there is an interesting clarification regarding the quantum of light:

Einstein’s theory of “light quanta” is not now generally accepted by physicists, but the argument above does not essentially depend upon their existence. All that is necessary is to assume that interchanges of energy between radiation and atoms can only occur in quanta. (Birtwistle 1926, 35–36)

If we remember that the book was written in 1926, this paragraph is somewhat surprising since, by then, the experiments of Arthur Compton had triggered a general acceptance of the light quantum.

Having established the existence, historical origin, and realm of application of the new theory, the rest of the book is an attempt to train students in techniques of quantization using a twofold strategy: to provide lots of examples where quantization is successfully applied, and to show that there is continuity between the methods used in “classical” and quantum theory. Because, as Birtwistle sees it, that is the only way one gets hold of the new physics: by using it, rather than by presenting it in a general form or analyzing its conceptual or philosophical implications. And this brings us to the main claim of this paper. Birtwistle, a first wrangler in the “Mathematical Tripos,” tried to teach quantum physics in the same way classical physics was taught in the Cambridge MT tradition: by repetition of examples, by solving specific problems, and by a relatively uncritical embrace of particular mathematical methods.

Once Bohr’s theory for the stationary states of the hydrogen atom has been introduced, the next step is to extend the quantum theory to more complex atoms. Here, he introduces Ehrenfest’s adiabatic principle, as a generalization of the Nicholson-Bohr quantum condition: “The question now arises, what mechanical entity is to be equated to for more complex systems than that of the hydrogen atom.” The answer was given by Ehrenfest who supposes:

[T]hat the “entity” which does not change under the influence of the slowly changing external forces must be an “adiabatic invariant” of the classical theory. This is the “adiabatic principle” of Ehrenfest, and it requires that only adiabatic invariants are to be equated to in order to determine the stationary states. (Birtwistle 1926, 41)

With the generalization of the quantum condition, Birtwistle embarks on a series of chapters explaining what he calls the basic “general dynamical theory,” chapters in which he fully shows his conditioning as a wrangler. The variation principle, 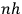Lagrange’s and Hamilton’s equations, the Hamilton-Jacobi differential equation and the ways to solve it, the Keplerian orbit, angle variables, and many other mathematical tools are explained. It would seem to be a book on mathematics (or classical physics) were it not for the fact that, at the end of some sections, the “quantum condition” appears. And it appears as purely the mathematical condition that some constant in the equations is equated to , without further ado.

As an example, we can pick chapter 9 on the Stark effect. After a very short summary of the effect, he says that “the classical theory fails utterly to account for the Stark effect,” and immediately develops the mathematics of Epstein and Schwarzschild’s solutions:

The dynamical problem to be solved is the motion of an electron due to a Coulomb center of force and a constant force parallel to a fixed direction. This is a particular case of two centers of force solved by Jacobi by the use of elliptic coordinates. (Birtwistle 1926, 97–98)

All this he explains from an exclusively mathematical point of view. At the end of the process, the quantum condition ( 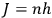) is imposed as part of a mathematical technique, through which the numerical results can be calculated and compared with experimental values. The reader is, thus, led to believe that quantum physics is in strict mathematical (and, therefore, physical) continuity with earlier physics, since the mathematical methods and formulas are almost the same.

It would be superfluous, in this paper, to give a detailed account of each chapter in Birtwistle’s book. The structure is basically the same for all: classical calculations in which the quantum condition is brought in as a particular mathematical trick that needs to be implemented to get a correspondence with experimental data. In 21 chapters, one can never find words such as “provisional,” “incomplete,” “failed explanation,” or anything that indicates that the quantum theory of the atom, as it is, might be viewed as incomplete or, worse, deficient. It is only in a rushed last chapter, written during what looks like his usual vacation in Norway,⁸ that Birtwistle introduces the reader to a list of unexplained phenomena like the anomalous Zeeman effect and the Paschen-Back effect, and to new theories, like the Bohr-Kramers-Slater theory (BKS) and the new quantum kinematics of Heisenberg. But there is no sense of stress, or crisis, or revolution. There are no value judgments. One gets the impression that everything introduced, even in these last chapters, is just steps in the development of the new physics.

Only in the last two pages, and in a statement that de facto undermines the whole project of this book, does he say:

Heisenberg has lately put forward the beginnings of a scheme of quantum-kinematics, which when more developed should lead to the direct deduction of these quantum theory formulae, without the intermediate use of the classical formulae in each problem considered. (Birtwistle 1926, 230–231)

This undermining of his entire first book leads us very naturally to Birtwistle’s second book, to which the next section is devoted. But before we move on, it is worth noting that Birtwistle’s introductory course in quantum theory was substituting for Fowler’s similar course from previous years. Actually, we also have a window into Fowler’s lessons, through Thomas’s complete classroom notes.⁹ Obviously, these notes have a spontaneity that Birtwistle’s book does not have, and one should compare the two documents only with caution; regardless, they show us very similar content (although with a sensibly different structure), but presented in a totally different style. Fowler was actively working on specific problems in the quantum theory and his lectures contain lots of qualitative explanations, experimental results, and a strong sense of the limitations of the current theory. It is, by far, much less mathematical than Birtwistle’s presentation, and mathematical developments go hand-in-hand with constant explanations of their physical meaning, something that is nearly absent in Birtwistle’s book. His style is closer to the old MT pedagogical system in which students were introduced to problem-solving techniques by repetition of cases. The aim of the lectures was seldom to challenge the status quo of the theory, but rather to give an account of how to use the accepted theory. And this is what, as I understand it, The Quantum Theory of the Atom is: a work to drill students in the quantization techniques, with very limited recourse to experimental results and with no critical outlook whatsoever on the limitations of the theories explained.

9.4 The New Quantum Mechanics

Birtwistle wrote a second book on quantum physics, related to his more advanced lectures on recent developments of quantum mechanics, the preface of which was signed in Copenhagen in October 1927.¹⁰ From a pedagogical point of view, The New Quantum Mechanics is very disappointing. Even in the respectful tone of an obituary, his biographer alluded to this fact:

Perhaps the least successful of his books was the last, on modern quantum mechanics. Here, owing to the novelty of the subject and the absence (when Birtwistle wrote) of other more systematic expositions (or indeed of any other exposition), the weakness of this deliberate method becomes more obvious. The book gives rather the impression of a collection of interesting isolated sketches. (Anonymous 1929, 881)

The New Quantum Mechanics is precisely that: a collection of the latest developments in quantum theory. In the words of another reviewer:

This account is very accurate and contains practically everything that has been done up to the summer of 1927. He gives us, so to speak, original abstracts of the principal papers and allows us a survey of everything that is known. This makes the work not exposition from one point of view, as is Weyl’s new book; it is rather an “impartial” treatment of the methods of the different schools, with credit given to each for its results. (Struik 1930, 32)

In Nature, Fowler spoke of The New Quantum Mechanics as one of the best examples of introductory books, an otherwise dangerous genre in the current state of affairs, in which Birtwistle gave “a convenient and faithful but uncritical reproduction of much of the earlier work of the theory” (Fowler 1929, 363).

The first five chapters of this book provide further examples supporting my claims at the end of last section. Birtwistle’s “impartiality” involves a neutral style in the sense that there are no critical analyses of the theories, or their theoretical or experimental limitations. These first chapters introduce the notion of spin, for which he needs to explain the problem with the anomalous Zeeman effect, the Stern-Gerlach experiment or Landé’s experimental formula. All of these phenomena were well-known long before 1925, when he wrote The Quantum Theory of the Atom. But none of these problems were mentioned in that book, except in the last chapter. Birtwistle was not training his students in the limitations and failures of a particular theory, but in its successes.

The matter-of-fact style is clear from the first sentences of the book: “The origin of the new quantum mechanics was an epoch-making memoir by Heisenberg which contained the new concept which was to lead to the phenomenal developments of quantum mechanics of the past two years.” And why was a new theory needed? “For some years before 1925, Sommerfeld, Heisenberg, Landé and Pauli had been grappling with the complex problem of the multiplets and their Zeeman separations,” which were only partly solved by introducing ad hoc half integers as possible values for the quantum numbers. Yet, again:

[A] real difficulty too had been met with in the spectrum of neutral helium, where two electrons revolve round the nucleus (the simplest many electron problem), all the theoretical results found being at variance with experiment; again in the problem of the “crossed” fields, where an atom is exposed to the combined action of electric and magnetic fields, fundamental difficulties arose. (Birtwistle 1928a, 1)

Obviously, in his previous book, Birtwistle never talked about these very “fundamental” problems, or about the limitations of the now “old” quantum theory, which was, at the time, the accepted way to solve those problems. It is only in 1928, after a new method has been found, that the limitations of the previous method are relevant: “Heisenberg’s new theory however at once led to the formula as the energy of the stationary state of Planck’s oscillator, so that half odd integers came quite naturally into the new results” (Birtwistle 1928a, 2).

In the last chapter, Birtwistle tried to summarize his understanding of the latest, as yet unpublished, developments coming from Bohr’s institute. Returning from his holiday in Norway, Birtwistle visited Copenhagen, but Bohr was not there, since the visit coincided with the 1927 International Physical Congress in Como, Italy. Thus, Birtwistle got only second-hand accounts of Bohr’s latest views. This was, however, one of the points that Cambridge University Press stressed in the advertising of the book. In an advertisement in Nature (1928), we read that the forthcoming book contains “new and hitherto unpublished speculations of Prof. Niels Bohr.” Certainly, the last paragraphs of the book include two footnotes, one referring to the meeting in Como, the other to the recent Solvay Conference. And, ironically, this was the source of the only research paper that Birtwistle wrote in his life: a note in Nature in which he qualifies the tone of the last chapter. There, we read that:

Prof. Bohr points out that the wording of the chapter may create the impression that these [probability] calculations were primarily developed in connexion with the new ideas [of complementarity], whereas they may be said to be characteristic of the whole recent developments of the quantum theory. (Birtwistle 1928b, 58)

Actually, the wording of this note was revised and changed by Oskar Klein and Bohr himself in Copenhagen.¹¹ This unfortunate anecdote demonstrates the limited understanding Birtwistle had of the depth of the new quantum mechanics and the conceptual, methodological, and philosophical debates around it, in spite of his relatively good mastery of the mathematics involved.

One last, revealing anecdote about the book comes from William McCrea, who was an undergraduate in Cambridge between 1923 and 1926. Talking about The New Quantum Mechanics, he recalled that:

[I]t was a remarkable achievement to produce such a comprehensive account of work newly published during the two years before the appearance of the book itself. Hartree described it to me in conversation as the “bare bones” of the subject, but it need not be only medical students who find it useful to have a skeleton for their studies.¹² (McCrea 1985, 58)

9.5 Conclusion

Contrary to Fowler’s or Darwin’s lectures, Birtwistle’s courses are seldom mentioned in the recollections of scientists who studied in Cambridge in the 1920s. That may be due to a number of different factors. It is possible that some bright students and future prominent physicists attended his lectures but forgot about them, influenced by the selective memory usual in these kinds of recollections. But it is also likely that Birtwistle’s courses were seen, already at the time, only as second best, as courses to be taken only by those wanting to get a feeling for the new theory, but not to master it and to work on quantum problems. Actually, in a letter to Dirac, Fowler admits that Birtwistle’s lectures are only meant for “complete beginners” who need “to get the ground work first.”¹³ That would explain why, among those scientists who became, in some degree, actors in the new quantum generation, we do not find students of Birtwistle (some of them actually remember his elementary lectures in mechanics and electricity, but not on quantum theory).

Birtwistle’s case can help us to understand another fact that is normally forgotten in the histories of revolutions. Quantum theory was not, for everyone, that revolutionary new theory that forced them into research. Birtwistle is an example of how one could, in times of change, stick to old methodological—not conceptual—paradigms. And, again, not all the students interested in quantum physics were necessarily potential participants in the forefront of scientific research. Having both Dirac and Birtwistle teaching advanced courses on quantum mechanics suggests that, as early as the late 1920s, there was room in Cambridge for a two-tier training system in the theory of quanta: one for potential researchers, another for people wanting only to be up-to-date with the latest science.

Abbreviations and Archives

References

Footnotes