4.1 Introduction
In this article I offer a reassessment of late antiquity and the Middle Ages in the history of mathematics. For this purpose, I develop a more general notion, of “deuteronomic” texts, i.e. texts depending fundamentally on earlier texts. I describe in detail some of the features typical to this period in the history of Western mathematics—late antiquity and the Middle Ages—where deuteronomic texts were crucial. I then argue briefly that those features had significant consequences in a changing practice, and image, of mathematics, and also that those features derive directly from the role of deuteronomic texts. Thus the argument is that ate antiquity and the Middle Ages had a real historical contribution to make; and that this derived from the basic nature of texts produced in this period.
Now late antiquity—and, largely speaking, the Middle Ages—did not fare well with the historians of mathematics. Pappus1—to take the most favorable case—is often considered the most competent mathematician in late antiquity, and Jones is his most careful contemporary reader. It is thus worth noting how Jones introduces his subject:
In the later Hellenistic period, after several hundred years of progress, the main stream of Greek mathematics, synthetic geometry, experienced a deep and permanent decline. The subject did not stop being studied and taught, but original discoveries became less and less frequent and important …
Pappus of Alexandria is the first author in this degenerate tradition of whom we have substantial writings on higher geometry [Jones (1986, 1)].
Wary of teleological readings of the past, many historians would probably react instinctively against such terms as “decline” or “degeneration.” Yet Jones’ judgment is inescapable. Something did happen at the end of the Hellenistic period, and “decline” is the term which comes to mind. My purpose in this article, therefore, is not to try to show how original late antiquity was—for it was not. It was deeply conservative. Yet, I shall argue, it still had a real contribution to make, if inadvertently: it developed a new project which differed qualitatively from that of early mathematics and which shaped the future history of mathematics. The paradox is that such a change came about without any intention, on the part of Late Ancient mathematicians, to change their mathematics, and my argument is that in certain circumstances, and especially inside mathematics, conservatism can act as a force for change (we shall need, however, to specify precisely the intended sense of this “conservatism” later on in the article).
So, to start, one should notice that Late Ancient texts often take the form of commentaries, and even when they are not commentaries they often are what I call “deuteronomic texts.” Late antiquity is the age of new editions, epitomes, and encyclopedic collections; later, during the Middle Ages—which, in this respect essentially pursue trends already discernible in late antiquity—another kind of deuteronomic text was added, namely translations—into Syriac, Arabic and Latin.2 The entire period from late antiquity to the Middle Ages is the age of scholia and marginalia. All such texts are “deuteronomic”: they explicitly start from an established text (or texts, in the plural), and aim at producing a new text, which reenacts the earlier text (as in a translation or a new edition), or uses it in more radical ways (an epitome or an encyclopedia). At least from the modern point of view, commentary is the most important kind of deuteronomic text, because it is also the most ambitious: it is the deuteronomic text standing on its own, apart from the original text; but in this article I shall stress commonalties among different kinds of deuteronomic texts rather than distinctions. In general, I shall see the commentary form as key to the understanding of deuteronomic texts.
Generally speaking, commentators are not highly regarded by modern authors, and they are often referred to by such pejorative terms as “pedantic” or “scholastic.”3 And once again, I do not wish to contest this characterization: my purpose is to identify in detail what makes an author appear “scholastic.” I shall then argue that such “scholasticism” may have real historical and philosophical significance and is, in fact, the vehicle through which conservatism can act as a force for change.
4.2 What is “Scholasticism”?
What do we mean by “scholastic” or “pedantic”? I shall now try to unpack such concepts with the aid of examples. I shall argue that there are several things we may mean by such terms, all closely linked. After we have seen some of these possible meanings, we shall try to investigate the possible link: what exactly do commentators tend to do, which earns them their pejorative epithets?
4.2.1 Scholia and “Vertical Pedantry”
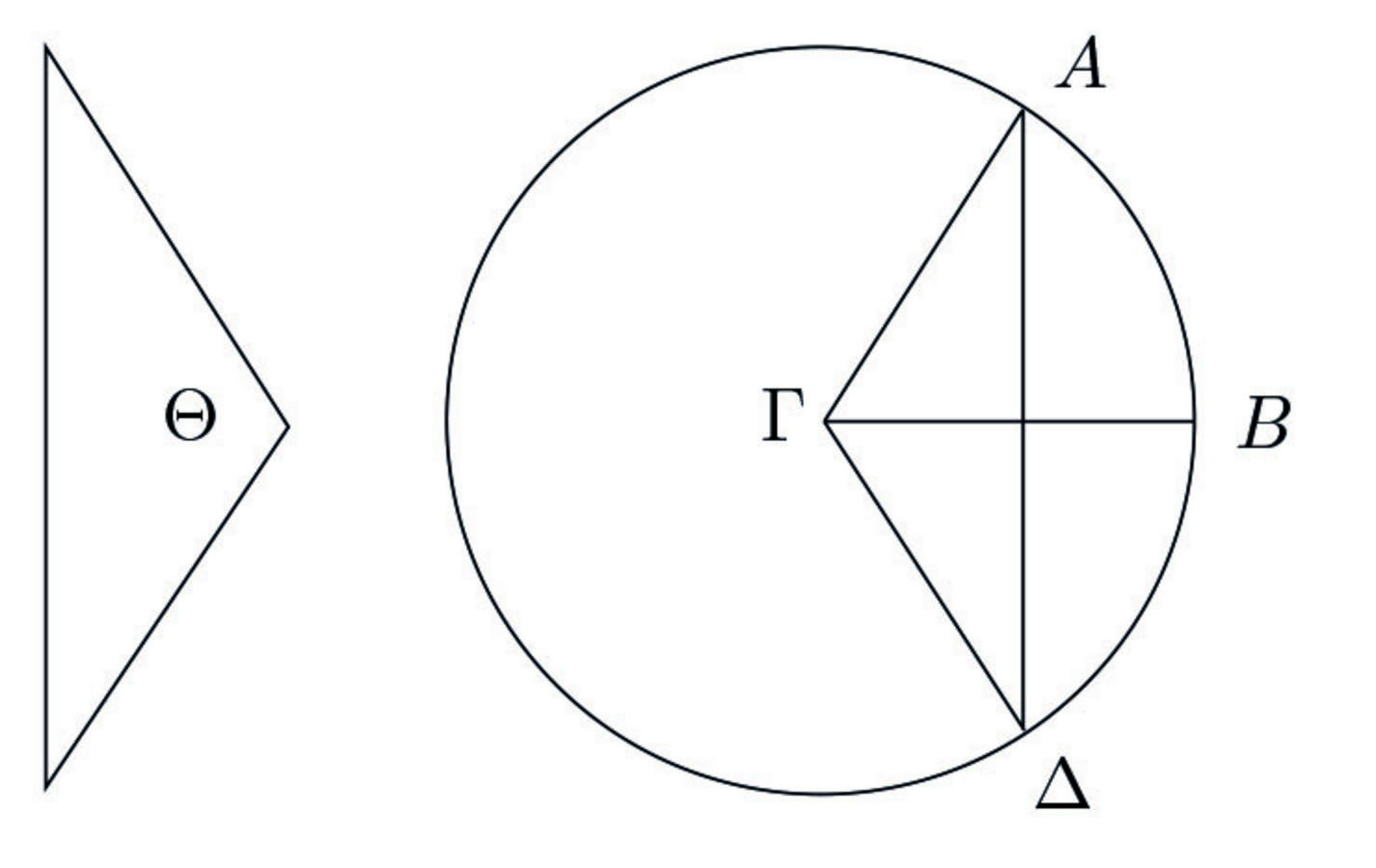
First, one thing commentators do is explain the obvious. This is vertical pedantry: they dig too deep. Of course, the “obvious” is difficult to define, and it is clear that shaped by its distinct mathematical education each mathematical culture will consider different things as “obvious,”4 but it is necessary to stop somewhere in a proof, otherwise Carroll’s well-known paradox ensues [Carroll (1985)]: in this paradox, to prove that Q derives from P you must prove that P yields Q, and then you need to prove that from P, and P yields Q, Q derives, and so on ad infinitum. This is not an empty philosophical worry: this type of regress may be called “the scholiasts’ regress,” and it is well-attested historically. Take, for instance, the final proposition of the first book of Archimedes’ Sphere and Cylinder. The following quotation has been taken out of the text itself (i.e. not from a separate commentary), but it is clear that Archimedes is not the sole responsible for this text. Scholia have accumulated and entered the Archimedean text, so that the deuteronomic text directly manipulates the original—a crucial point to which we shall have to return. This is how it works explicitly (Figure 1).5
[A conclusion of Archimedes’ line of reasoning:]
Therefore the figure inscribed in the sector, too, is greater than the cone Θ; which is impossible.
From the mathematical context, impossibility can be seen directly: in fact, it derives immediately from the proposition just preceding this one. To note this fact, some scholiast added the following comment:
for it has been proved in the [proposition] above that it is smaller than a cone of this kind.
This is the first pedantic note, the first explanation of the obvious. Now the same scholiast, a minute later, or another one, a century later, hastens to add:
that is [a cone] having [as] a base a circle whose radius is equal to the line drawn from the vertex of the segment to the circumference of the circle (which is [the] base of the segment), and [as] a height the radius of the sphere.
This is of course the standard description—scholiasts care about standard descriptions, a fact to which we shall return. But wait – is this really the cone we have here? Yes, assures the scholiast:
and this is the said cone Θ.
Or is it? Wonders the same scholiast, or yet another one, yet another century later: We must say why!
for it has both: [as] a base, a circle equal to the surface of the segment, that is [equal] to the said circle, and a height equal to the radius of the sphere.
This is, then, what one calls “pedantic”: explaining explicitly what should be understood implicitly—a process to which there is in principle no end and which therefore can make the pedant look not only dim- witted, but also absurd. At any rate, this is clearly one type of pedantry, vertical pedantry: digging too deep. Apparent absurdities of the type quoted above are relatively infrequent (though more can be easily added: e.g., in the same book, Proposition 13, [Heiberg (1910, 56–24)], because scholia become recursive only when an original scholion becomes part of the transmitted text—a common but far from universal phenomenon; while marginalia to marginalia are less common. However, this is almost the most common type of scholion we find in mathematical works: a brief, essentially trivial, mathematical explication, showing why a derivation works—a question which in principle could always be raised and therefore was less frequently raised by the original Greek mathematicians.6
4.2.2 “Horizontal Pedantry”
So far I have described what I call “vertical pedantry,” where you dig too deep. Another, related type of pedantry is “horizontal pedantry”: digging too wide. Just as one can go on proving obvious things, anterior to the proof, so one can go on proving implied things, posterior to the proof. From the point of view of both classical Greek mathematics and of modern mathematics, such proof of implied results may seem redundant. A proof, certainly a classical Greek proof, typically deals with one single case, and leaves several other ones merely implied. Commentators, then, often go on to prove these implied cases. Take Figure 4.2 as an example: this is a lemma proved (probably) by Archimedes and quoted by Eutocius7 in his commentary to the second book of the Sphere and Cylinder. The lemma shows that on line AB, a certain maximal area is obtained at point E. This is shown by taking another arbitrary point Σ, and showing that the area obtained by it is smaller than the one obtained by E. This is because a certain hyperbola is always, in the area above the line AB, “contained” by a certain parabola, and in such a way that these conic sections are tangent at point K, just “above” E. Thus the result is implicitly seen to be general: since the point of the tangency is unique, the area obtained at E must be maximal. Such implicit generality is typical of classical Greek mathematics, indeed of mathematics in general: you take an arbitrary case and show a certain result, and since the grounds for the result are seen to be general it becomes immediately clear that the same result is obtained in general, and not only for the arbitrary case taken for the sake of the proof. So this is a general proof, taking Σ as an example, where nothing in the proof relies upon taking Σ on this side of K and not the other.
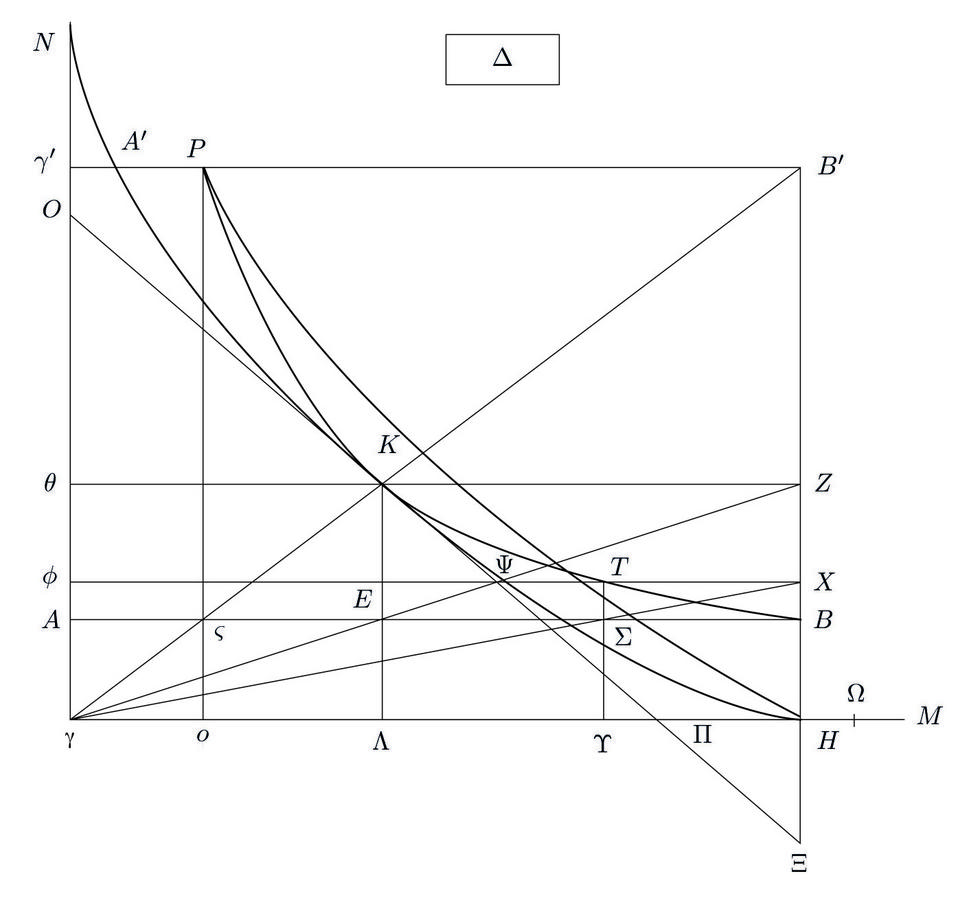
Fig. 4.2: Reproduced with kind permission of Springer-Verlag.
The above, however, only is one part of the proof as it reached us. As it stands in the available text, following this first part, the proof goes on to address the special case of another arbitrary point, ς, on the other side of E. In Netz (1999a), I have argued that this second part is an addition due to Eutocius. So, Archimedes produced a general proof that only considered the case right of point E, and the commentator Eutocius inserted a completely redundant proof for the left side of E. This is horizontal pedantry, literally horizontal in this case. You dig too wide. You go on proving what has already been implicitly proved, by adding cases that actually are no more than trivial extensions of cases already considered by the original. Notice that, if my interpretation is correct, the commentator has once again manipulated, and intervened on, the text—just as we saw with the accretion of scholia in Archimedes’ Sphere and Cylinder. This, of course, was not done in the intent of misleading—I believe Eutocius expected his readers to recognize the point where Archimedes’ proof ended and his own appendix began. But he in essence produced a sequence of texts, partly authored by Archimedes, and partly his own, and later readers were led to take Eutocius’ special case as a part of Archimedes’ own proof. As an historical figure, Archimedes was therefore partly constructed by Eutocius.
While the example mentioned here is special due to its great complexity, it nonetheless is very typical on other counts (which obviously is a necessary part of the argument developed in Netz (1999a)). Commentators and other deuteronomic authors do often pile up cases. For example: Brentjes (1998) has analyzed the main additions to Euclid’s Elements I in Arabic translations/editions. Of the eighteen clusters of additions dis- cussed by Brentjes (we use the term “clusters,” for those additions often differ substantially from one line of tradition to another), 14 involve such case-additions, in which, Euclid having proved one case out of several possible ones, the Arabic and Latin authors (or their Arabic, or Greek sources), added further cases either in a commentary, or inside the translated, viz. edited, text itself. To pile up more examples always repeating the same pattern would be unnecessary. In every example as in the above, the main form of addition to a given text—the main form of deuteronomy—was the addition of cases.8
4.2.3 Standardization
Besides adding trivial proofs, there are other ways by which commentators revealed their “scholastic” nature, such as drawing attention to allegedly trivial aspects of the text, such as form versus content. Of course, the word “trivial” may be misleading, since it will be obvious that form is not unimportant in a subject-matter like mathematics. Still, we are here facing the same principle: a deuteronomic author pays special attention to what may have been of lesser importance for the original author. While the latter cares mostly for mathematical facts and proofs, the former delves on the form in which such facts and proofs are presented. This has at least two levels.
First there is the level of the local form, that is the form of the individual proposition. Commentators devote time to describe this specific form, to refer to it in various ways. It must be understood that the very vocabulary we have for this is an invention by commentators. The Greek terms for the parts of the proposition are familiar: protasis, ekthesis, diorismos, kataskeue, apodeixis, sumperasma.9 Here is how Proclus describes this,10 “every problem and every theorem that is furnished with all its parts should contain the following elements: an enunciation, a setting-out, a definition of goal, a construction, a proof, and a conclusion.” This is one of the most well-known and influential passages in Proclus. What must be stressed is that Proclus himself most probably invented these terms and this analysis. Not a series of terms used by the classical Greek mathematicians, these are rather specific to commentators’ ways of speaking; they betray an interest in the description of form, which is typical to commentators.11 It is also very typical that, starting from a practice to a great extent already present in Euclid’s own text, Proclus’ description goes beyond the actual systematizing present in it: this is a typical process of canon-formation, where the granting of canonic status goes hand in hand with the transformation of the original text. Even more pedantic is Proclus’ actual claim that these forms should be present: he wants to standardize the form of mathematical texts. Now, at this point, Proclus’ commentary is part of a commentary on the first proposition of the Elements. In this connection, it is therefore interesting to consider the text of this proposition as printed by Heiberg, in his standard critical edition. The end of the first proposition includes a stretch of text in square brackets, i.e. belonging to a textual tradition that is rejected by Heiberg. The text runs as such:
Therefore an equilateral triangle has been set up on a given limited straight line [Heiberg (1883, 12–17)].
Heiberg’s critical comment reads: “omitted by all the manuscripts; from Proclus only; accepted by August; hardly could be genuine.” (“From Proclus” refers to the fact that Proclus, who quotes extensively from the text, quotes this sentence, too, as if it were in his text of Euclid.) While Heiberg rejected this sentence, other editors have however accepted it, and followed Proclus instead of the manuscripts. But what is the text? It is the conclusion—the final part of the proposition which according to Proclus should appear in every proposition. It is almost certain that, while commenting on the first proposition and in this context introducing his general analysis of the desirable form of propositions, Proclus changed the text, so that it fits his own schematic system.12 He added a part not present in Euclid’s original text, simply so as to make Euclid comply with his formal stipulations—and this addition has later become part, not mainstream admittedly, but still part, of the Euclidean tradition.
This case hardly was isolated. According to Proclus, the enunciation should for instance be repeated verbatim in the conclusion, and this is generally the case. But there is evidence that, when faced with conclusions deviating from enunciations, later scribes sometimes modified them. Indeed, in some but not all manuscripts, disagreements are to be found between enunciation and conclusion. Whenever such disagreements are not obvious scribal mistakes, a lectio-difficilior type of argument makes probable the hypothesis that they were already present in the archetype, and that in some manuscripts either the enunciation or, more probably, the conclusion were corrected so as to agree with the other.
As one gets further away from original texts, such formal awareness of proposition structures becomes increasingly striking. In many translations of Greek mathematics, there is a tendency to signal the various parts of the proposition in standard ways, to introduce the proof, e.g., with the words “proof of that,”13 or to label the various parts of the proposition (or at least some of them) by explicit titles such as “demonstratio,” “conclusio,” etc.14 As a result of an analysis devoted to the form of individual propositions, a general standardization process was therefore undertaken by late commentators.
4.2.4 Classification
Beyond standardization, this attention paid by deuteronomic authors to the various parts of propositions manifested another of their tendencies, namely to offer metamathematical terminology and classification. Other forms of this tendency had a more direct mathematical significance. In this context, probably, was for instance developed a classification of geometrical solutions in antiquity: “planar” (i.e. requiring only elementary Euclidean methods), “solid” (requiring conic sections), and “linear” (requiring some specially generated lines).15 While the accumulation of mathematical solutions from antiquity suggests that authors were happy to push ahead with whatever means they had at their disposal, later authors show a more restrictive approach. Pappus for example criticized a suggested solution for failing to belong to the correct class. This demand of Pappus—that solutions should belong to certain correct classes—is analogous to the demand of Proclus mentioned above about the arrangement of propositions in certain, correct parts. While the classification of kinds of solutions has, in principle, a sound mathematical basis, this mathematical basis could not be proved by ancient means. The Greeks simply did not know the mathematical relations between the various types of solutions: all they had, as a basis, was the corpus of accepted solutions. The classification of solutions relied, therefore, on the authority of a mathematical canon: a typical “scholastic” use of tradition.
4.2.5 Systematization
We have examined above one level of attention paid to formal structure, namely that of the form of individual propositions. At a more global level, there also is a similar “formal” attention. Here we definitely begin to approach some very deep transformations effected by commentators. One especially significant example will be introduced here.
Book II of the Elements was at the heart of the controversy surrounding the so-called geometrical algebra.16 The book proves geometrical relations which may be considered as giving solutions to basic algebraic equations. Unguru and others have argued that this is not algebra, for two main reasons. The first point is this: there is no attempt to derive one equation from another, to show the interdependence of the equations—which would have been very natural if you were to approach them as equations. Each proposition is proved separately and there is no tight deductive structure—in fact, this is one of the least deductively organized books of the Elements.17 So what are the proofs based upon? Here comes the second point: each of these propositions is proved, separately, through geometrical relations holding only in the specific configurations of a given proposition; everything is done geometrically, through the diagram. This is how Euclid’s Elements, Book II looks like, and therefore it is geometry and not geometrical algebra.
But then, it was transformed by a later tradition, and I do not refer just to modern historians of mathematics. An Arabic commentator to Euclid who died c. 922, Al-Narizi reports a comment of Hero’s in which alternative proofs are offered to most propositions.18 Since seen from our distant perspective they seem to belong to the same project, we need not go into the question of distinguishing what in Al-Narizi’s text is due to Hero or to Al-Narizi. But what exactly is this pro ject and what are the alternative proofs reported in Al-Narizi’s text? These proofs have two main features: (1) since a single chain of deduction unifies the book, each proof refers back to earlier ones; and (2) as a result, proofs are less dependent on diagrams. Hero and/or Al-Narizi have taken a series of self- sufficient proofs and turned them into a single unity. This is an example of global formal systematization, and here we see very clearly that the entire nature of the work has been changed. It is still premature to call this algebra, since the intention is still spatial; yet something deep has been changed. This text, one must emphasize, bears no trace whatsoever of any philosophical consideration underlying the transformation. Al- Narizi/Hero did not set out to be as deductively unified as possible (not a feature of Hero’s own work). Moreover, in other parts of the commentary on Euclid, Al-Narizi/Hero still heavily relied on diagrams, just as Hero did in his personal work. Simply, Al-Narizi/Hero tried to introduce a global organization into a work originally very discrete in structure. The goal is to add cohesion and systematization of which the text seems to be lacking. Such types of unification therefore are on a par with the transformation introduced by the scribes who have changed conclusions to fit enunciations. It is on this level of “scholastic,” formal detail that Hero and Al-Narizi work. Yet, we begin to discern the possible significance of these transformations. Not surprisingly, to add cohesion and systematization clearly is a significant transformation.
There are many other ways in which deuteronomic texts are more systematic than the originals from which they start. One typical process occurred with the text of Ptolemy’s Almagest. Our text can be shown to derive from a Late Ancient recension, one feature of which is the systematic insertion in the text of titles at the head of each chapter, and summarized at the beginning of each book [Toomer (1984, 4–5)]. It should be realized that in all probability Ptolemy’s text totally lacked chapter divisions (not an Ancient practice), let alone chapter headings. Whereas the original Ptolemy was a discursive text that proceeded smoothly and without breaks through the entire astronomical corpus, the Late Ancient tradition created a Ptolemy progressing from one discrete, well-signaled point of argument to the next. Once again, this process is typical of a type of canon formation which transforms the original. Briefly, the Late Ancient tradition created a more explicitly structured Ptolemy, not by changing the nature of his astronomy, but merely by adding to his presentation of this astronomy.
4.2.6 The Phenomenon of Epitome
Yet another kind of global systematization is the epitome, one of the main forms of deuteronomic texts (or of canon formation): a new edition whose main feature is to abridge the original. This gives rise to a certain paradox, as noted recently by Vitrac19: while late editions often add to the text (this is the gradual accretion of scholia and marginalia, noted above), they may also subtract from the text. This important phenomenon is worth a detour.
Many Arabic and Latin manuscripts of Greek mathematics take the form of abridgments, a fact which is not often noted, since those abridgments are not used for the printed editions (which rely, instead, on fuller versions). But the printed editions give in this respect a misleading sense of the nature of the world of manuscripts: this often consists not of complete versions, but of abridgments. Sometimes the abridgments form distinctive editions, which may even be the only extant versions: for instance, Archimedes’ Measurement of the Circle is extant, already in the Greek, only in such an abridged form.20 Most important, it is known from Proclus’ commentary to Euclid that a certain Aigeias (?) of Hierapolis has prepared, in ntiquity, an epitome of the Elements.21 We are told that Aigeias combined together two different propositions on parallels, that are Propositions 27 and 28 of book I. In principle, such a procedure could result in a monster-proposition having a double proof, dealing separately with each of the conditions. Another possibility, perhaps more natural in an epitome, is that Aigeias did not offer any proofs at all, but provided his readers, instead, with an abridged version of the Elements consisting of the enunciations alone: at the level of the enunciation, such a combination of Propositions 27 and 28 is indeed natural.22 It is of course impossible to say whether this is the case, but it is clear that such epitomes (which are common in our manuscript libraries) circulated in antiquity. There are four known papyri surviving from antiquity which can be considered to derive from a “text” of Euclid, in some sense.23 Of these, P. Mich. iii.143 only contains definitions, and therefore could belong to a “complete” or “abridged” text—if to a text at all (and not just to a private memorandum).24 P. Fay. 9 most probably belongs to a complete text of Euclid (though different from our established text).25 Dating from the second century AD, P. Oxy. i.29, already is different: it contains no letters inside the diagram (which the text of the proof would have required) and we happen to have only the enunciation of Proposition II.5 (immediately following the conclusion—or the enunciation?—of Proposition II.4). It was therefore conjectured by Fowler that this is not a “complete” text of the Elements, but some abridgment containing only the enunciations; this conjecture is now corroborated by a recently published papyrus, P. Berol 17469 [Brashear (1994)]. Also dating from the second century AD, this papyrus contains the (unlabeled) diagram and enunciation of Elements I.9, surrounded by tiny remnants of the same for Elements I.8, 10. Thus it must come from a similar abridgment which gave only enunciations and unlabeled diagrams.26 Thus, while the archeological evidence is very slim indeed, it is also, after all, the most direct evidence we have, and it shows that, at least by the second century AD, the Elements circulated not only in the “full” Euclidean form, but also as an (Aigeian?) epitome. And this has an immediate significance, for it is clear that such a text of the Elements has a meaning radically different from that of the complete text: that is, it is no longer a set of mathematical explorations, efforts to show the truth of mathematical claims; it becomes a static repository of mathematical results, whose truth is taken for granted just on the basis of trust in an author. The interest now is no longer in getting to know why (i.e., getting to know why such and such mathematical claims are true), but in getting to know what (i.e., what Euclid has asserted—which is also taken to represent what the only mathematical truth is). Once again, this is a “scholastic” transformation: from critical exploration, we move, perhaps, to a more passive absorption of established results. So while epitomes are the opposite of scholia, in that epitomes subtract where scholia add, epitomes are also akin to scholia in nature: both, I suggest, result from an attempt to get to a text which will stand as a perfect repository of the truth on a given subject, and both represent what may be considered a more “scholastic” cast of mind.
4.2.7 Correctness
There are further senses of “scholastic” or “pedantic,” which we need to unpack. For instance, I have mentioned above one way in which commentators care for allegedly trivial things; namely that they care for form rather than for content. Moreover, they may be said to care for “correctness” rather than “intelligence”: that is, an adherence to values which are projected into the canon. This is related to the effort to standardize things, to the interest in form. Proclus is almost like a schoolmaster, who praises his pupils especially for their neat handwriting. He often gives marks to Euclid, almost always for logical correctness, never for the brilliance of ideas behind the proofs. I have found four places where Proclus was moved to express real emotion concerning details of mathematical practice, saying that he “likes” this or is “amazed” by that,27 and they are all about precision—e.g. that Euclid is making all the necessary qualifications, or is having the proof fit for all cases (again the interest in cases!). It is interesting that this is what Proclus gets excited about.
4.2.8 Consistency
I wish to concentrate on a very special kind of “correctness,” namely, consistency. Deuteronomic authors often introduce consistency—e.g., as we have seen already, they may “correct” the text where it diverges from patterns such as the Proclean parts of propositions. A special example of such consistency may be seen with Euclid’s Optics. While the philological problems involved in this case go beyond the scope of this article (and are by no means completely solved), the picture which seems to emerge from recent studies is as follows:
1.We have two versions for the so-called “Euclid’s Optics,” which may be called version A and version B.
2.Heiberg thought that version B derived from version A [Heiberg (1895, xxx–ff)].
4.This is a typical situation in text transmission: albeit closely linked, no version can be shown to have been derived from the other. So, they most probably represent two separate transformations of a lost archetype.28
Assuming therefore that version B is a post-classical transformation of a classical original, one may note with profit that it exhibits a very interesting kind of consistency. It is the only Greek mathematical work in which a letter is very consistently attached to an object: the letter K to the center of the circle29: of the 24 centers of circles, 12 are denoted by K, while the remaining 12 can be seen to be part of diagrams closely related to those in version A (where, for comparison, there are 22 centers, out of which only one is denoted by the letter K). So version B introduced a new kind of consistency, not known before in Greek mathematics: the letter K, by being consistently used, acquired a meaning as a symbol (and not merely an index referring to a point in the diagram): regardless of the diagram, K “meant” a center.30 I believe that version B’s author wanted to produce a more correct text by adopting such usage. He made sure that the same object was consistently referred to in the same way. This is of course speculation piled upon speculation: it is impossible to prove that the K -convention is deuteronomic, and it is impossible to be certain about its origin. But while speculative, this is also the simplest explanation: a deuteronomic author, caring for consistency and “correctness,” obtaining, inadvertently, some far-reaching results.
4.2.9 Erudition
Finally, yet another thing which commentators care for is erudition.
Instead of understanding itself—just reading something and understanding it—they display their erudition, that is, their understanding of how the text may be situated in a wider context of other texts. There is an obvious way in which this can be done in mathematics, and in fact here is one of the great innovations of commentators. They add references to previous propositions, which supply the grounds for the mathematical claim. I attach a figure showing a typical page from a Byzantine classical mathematical text (Figure 3).31 In this example, while the main body of text reproduces the classical text, which of course has often been transformed in the various ways I have described above, margins contain scholia introduced by Late Ancient commentators. On the right-hand side are more detailed mathematical scholia. On the left-hand side, there are two very brief marginalia which state respectively (typically, using a shorthand): “through a reductio argument” (another example, therefore, of scholiasts being interested in classifying their material according to some metamathematical scheme), and—what I wish to draw attention to right now—“through the 5th proposition of the 10th book.”
There is nothing corresponding to this last statement in classical Greek mathematics, where you just understand, directly, the proof, based on your internalized toolbox of mathematical results.32 You do not state explicitly which earlier book you may be referring to, and most probably you are unable to recall this—you will be unable to cite chapter and verse. You just know the fact, not the textual reference. This is understanding, which commentators replace by erudition: they supply chapter and verse reference and, for this purpose, they do a mighty thing: they introduce chapters and verses, the key to mathematical canon formation. Euclid may be an exception to this (again, based on the papyrological evidence) but other classical authors, such as Apollonius and Archimedes, probably did not give numbers to their propositions—which is shown by the fact that different manuscript traditions, as well as different commentators, adopt different numbering systems (often included in the margins of propositions, as any other marginal comment). This situation may be compared to that of chapter headings, such as those introduced in Ptolemy’s text. Late Ancient readers introduced, moreover, references based on these numbers. As the numbered proposition is among the most prevalent features of the format of contemporary mathematics—without which we can hardly imagine a mathematical textbook!—it should be obvious that the kind of mathematics that is done with this practice is different, in a significant way, from the one done without it.
We have now compiled several significant transformations introduced by deuteronomic authors in classical texts; it is time we try to appreciate the overall significance of these transformations.
4.3 “Scholasticism” and Deuteronomic Texts: Their Historical Significance
We have reviewed above a number of interrelated phenomena. First, there is what I called “digging too deep” (a) and “digging too wide” (b), that is, respectively, the phenomenon of proving the obvious (adding further arguments in order to fill out the proof with details that were left out by the original mathematician, and therefore obtaining a complete chain of proof ), and the phenomenon of proving the implied (adding in all cases, so that the proof actualizes all conceptual possibilities and covers them all). The result of both these processes is a text seen as aiming at becoming a one-to-one map of the conceptual world it captures.
Next, we have examined the introduction either of a brand of standardization that took the proposition as the textual unit (c), or else a similar kind of systematization of text structures at a larger scale (e). Many processes are related to such standardization: introduction of greater consistency, for instance in the use of symbols (h), or display of erudition, especially textual erudition (i). All of these processes have to do with a great attention paid to the text as text: attention is focused, not so much on the world captured by the text, as upon the text itself. The important thing is no longer simply to provide a valid proof, but also (and perhaps even more importantly) to display it in a correctly organized text of the proof. The basis for statements is no longer mathematical validity alone, but also a reference to some specified earlier units of text.
Related to the above is an interest for metamathematical features that may have seemed less important to original authors—but which are constantly referred to, as if they based on the accepted canon’s authority. No one explicitly states that works by, say, Euclid, Apollonius, and Archimedes are to be considered “canonical,” but the very fact that these, and no other, works are transmitted produces a new significance for them. Texts are important, not only for what they say, but also for the very fact that they are there—they come to represent mathematics as it was available to Late Ancient and Medieval cultures. This has several consequences. Most important, there is now a stress on more formal “correctness”—given by the canon as interpreted by, say, Proclus—instead of on mathematical originality as such (g); much freer in the original, the possible range of practices gets pigeon-holed and delimited by prescriptive rules, so that the mathematical enterprise becomes not so much the discovery of new results—hitherto unknown facts—but the presentation of known facts along prescribed rules (d); finally, epitomes create a new type of mathematics: not a set of discoveries which the reader is expected to react to and criticize, but a set of accepted results which are solely validated by their status of canonical texts.
To sum up, then, there are three main vectors involved in the “scholastic”: the attempt to construct the text as a one-to-one map of the conceptual world it refers to; a focus on the properties of the text as text; and the introduction of a more limiting, prescriptive code of mathematical practice, invoking a canon (which is constructed and manipulated for this purpose), so that mathematics gets to be defined in part, I suggest, simply by its adherence to this canon.
What does all that mean in terms of the image of the mathematical practice?
First of all, it is important to stress that all this has to do with mathematical practice. While the authors I describe have a special fascination with texts, they are not philologists. They do not engage with mathematical texts, as a modern textual critic does, in order to say something about texts, hat is about the history of pieces of writing. While texts are the focus of attention, they are the focus of mathematical attention: an attention to mathematical texts is perceived as the way to do mathematics. It is only after textual criticism comes to be established as a separate activity, with its own goals and criteria – in the XVIIth or even XVIIIth centuries—that Western mathematics cuts its umbilical cord to the Greek canon; but up to this point, reference to the Greek canon simply was mathematics. This is not to say that this culture stressed philology at the expense of, say, mathematics; no, it rather is that mathematics itself saw its image shaped by references to a textual canon. The three vectors—the one-to- one map between text and conceptual world, the interest in texts as texts, and the role of the canon—all influence the way mathematics is done.
Furthermore, these three vectors influence mathematics in a clearly defined way. While these three vectors may point at somewhat different directions, so that, sometimes, “scholasticism” may lead to opposite results (as noted above, following Vitrac, for additions and subtractions to the texts), those three vectors, taken together, do imply a coherent image of mathematics. Let us try to put together this image in greater detail.
One central feature of this image is that it involves the idea of a perfect text. Of course commentators are well aware of the fact that the actual texts in front of them are not totally perfect: not all arguments are made explicit, not all cases are set out. But this very recognition of defectiveness implies the possibility of completeness. What Euclid elides, is something which in principle he could have added in, so that in principle—and as an aspiration—one can think of a perfect mathematical text.
Standardization implies, again, that there is a standard—that there is one unique preferred way of presenting mathematics. And while the perfect, unique text is not identical with the actual canonic texts, such as Euclid, the actual canon is often taken as a standard, and the very existence of a venerated canon further strengthens the image of mathematics as striving towards an ideal text.
This ideal text is also unified. It is of course all standardized, and completed in a logical sense; it is also tied together in a rich inter-textual web constituted by the internal references introduced in the canon. What original mathematicians had produced as isolated, ad hoc solutions to problems, becomes part of a single system of interdependent propositions, all embedded inside a single canon. Thus an image is projected, of a unique, ideal Mathematics—now with a capital ‘M.’
Is this ideal Mathematics a conceptual, or textual, object? In a textual presentation aiming at an ideal one-to-one map between conceptual world and text, this question becomes blurred. While original texts are discursive, and present ways of thinking about a mathematical problem or situation (which are only referred to through the text), the new mathematical canon, at least ideally, may mirror everything inside the text itself. Thus the image may be defined as that of a unique, perfect, text-like Mathematics. This is the image of mathematics as constructed by “scholastic,” deuteronomic authors. If we are able to unpack very easily the contents of such an image, this is largely because this image is still with us; but I claim that such an image did not exist in classical antiquity.33
In other words, “scholastic” authors approach mathematics as text, and construe it as text; and therefore they reinterpret the mathematical past as so many steps in the direction of constructing the perfect text. Proclus’ account of the origins of the Elements is typical. In a very well- known passage on early Greek mathematics, Proclus describes a progress, where Euclid’s Elements are gradually put together and re-edited by the entire sequence of mathematicians up to Euclid himself. Hippocrates of Chios, Leon and Theudius of Magnesia are explicitly said to have written earlier versions of the Elements; other authors are described as “adding results to the Elements” or “perfecting,” “arranging better,” previously known results, until finally:
came Euclid, who brought together the Elements, systematizing many of the theorems of Eudoxus, perfecting many of those of Theaetetus, and putting in irrefutable demonstrable form propositions that have been rather loosely established by his predecessors [Friedlein (1873, 68–10)].
Yet clearly the line of historical development was in all probability radically different: people in the Vth and IVth centuries BC were not preparing the grounds for Euclid, but were pursuing their own pro jects (which are only vaguely understood by us). It is far from clear that anyone prior to Euclid has tried to put together a book of Elements. Proclus has simply looked at the past through the spectacles of the mathematical practice he himself knew, so that he perceived mathematicians as aiming towards an ideal, unique text—the ultimate goal.
Five centuries later, Al-Nadim, the author of the Fihrist—an early biobibliographical study, i.e. a deeply deuteronomic text, most clearly expresses the same position34:
Euclid, master of Geometry.
He was Euclid, son of Naucrates son of Berenicus, and he was the discloser and proclaimer of geometry, preceding Archimedes and others.
Here finally Euclid—which, especially in the biobibliographic context of Al-Nadim, means the text of Euclid—becomes geometry personified. The religious overtones are perhaps not accidental, and it is immediately obvious that the same connection between deuteronomic texts, canon formation, and the ideal of a perfect text, can be found elsewhere in the cultures of late antiquity and the Middle Ages. But while such comparisons are meaningful, I will argue that there is a special story to be told specifically about mathematics. I therefore proceed to offer my suggested principle in the history of mathematics.
4.4 Conclusion: Deuteronomic Texts, a Suggested Principle in the History of Mathematics
My argument is embarrassingly simple. Because, in late antiquity onto the Middle Ages, deuteronomy became the main form of doing mathematics (and, as explained above, not just a way of working with mathematical texts), and simply for this reason, mathematical practices came to the fore which were marked by this second-order context of texts referring to texts. The center of activity became the correction, correlation, and manipulation of an established canon of texts. Commentators perfected proofs, added references, produced epitomes and so on—what else? They do not write directly about circles and triangles, what they write about is about writings about circles and triangles, and therefore it is a tautological result that what they write is a writing about writing.
What makes this story more interesting than just a tautological account of the way in which second-order texts are indeed texts about texts, is the fact that we can see here a causal mechanism, starting from more general cultural forces, and leading to a special result inside mathematics. I shall not attempt here an explanation of the forces which made late antiquity and the Middle Ages what they were, but (as already suggested briefly in the context of Al-Fihrist), these periods are characterized, in general, by the production of deuteronomic texts. Perhaps this has something to do with cultural forces such as the growing role of scriptural religion in these periods; perhaps this has to do simply with the inner logic of the proliferation of books leading on to canon formation and to the growing role of deuteronomic texts positioned relatively to such a canon. At any rate, deuteronomy could probably be found everywhere: in Jewish law as in Greek philosophy, in Arabic grammar as in church doctrine.
But while this process is universal—and thus may of course have universal consequences—it necessarily effects mathematics in a special way, because of its special conceptual nature. As has been pointed out by Corry (1989), mathematics (as well as, to a lesser extent, philosophy) is the one discipline where first and second-order discussions are part of the very same discipline. For instance, the question whether such and such proposition is provable on the basis of such or such tools is a mathematical question. Being essentially about arguments and reasons no less than about facts and objects, mathematics is extremely sensitive to shifts toward the second-order. The moment you begin asking questions about mathematics, you thereby begin asking new questions inside mathematics itself. Thus the mathematics where you clearly distinguish he parts of the argument (as Proclus insisted on), or in which you attempt to classify legitimate solutions to problems (as Pappus insisted on), is a different mathematics from the one in which you are simply giving arguments in order to try solving a specific problem in whichever way you can. In particular, once the image of mathematics becomes that of a perfect, unique, text-like object, this gives rise to new pro jects: for instance, attempts to remove blemishes from this ideal object (hence, for instance, the history of the parallel postulate35), fill gaps in the structure of the canon (so, for instance, Al-Haytham’s completion of the Conics—which has so many early modern parallels36) or to produce total solutions, which will exhaustively cover the whole conceptual space (for instance, Al-Khayyam’s algebra, as well as of course many strands of the scientific revolution). All of this, I argue, is a direct result of the intervening culture of deuteronomic texts, which changed the image of mathematics—how could it fail to do this, being essentially second-order?—and which therefore paved the way for modern mathematics.
Two caveats, finally, are necessary.
First, while I offer here a speculation on how certain changes emerged in the image of mathematics from antiquity to late antiquity and the Middle Ages, in this way “reducing,” in a sense, images of mathematics to its textual practices, I am of course neutral as to the validity of such images of mathematics. To say that an image has arisen historically is not to say that it is either true or false. Perhaps is it more correct to see mathematics as an ideal, unique, text-like object; perhaps is it correct to see it as a set of more isolated, ad-hoc practices; perhaps, finally, is this entire question of “correctness” misguided here: I have no view to offer on this question.
Second, while I argue for a direct causal role for the very fact that those texts were deuteronomic, I avoid trying to delimit the possible ways in which the presence of deuteronomic texts may effect the practices involved in a given cultural domain. It is obvious that such effects are determined partly by the nature of the canon itself. The Greek mathematical canon was characterized by the presence of logical arguments, and it is therefore natural that deuteronomic texts based on this canon would focus on this aspect and transform its meaning. In other domains or cultures, the logical proof need not have been so dominant in the original canon, so that deuteronomic texts would have different effects: one may consider the case of Chinese mathematics (where commentaries are no less important as vehicles of mathematics), and it seems that, in this case, deuteronomic texts transformed mathematics in a different way.37 From this point of view, my aim here just was to offer one possible line of development in what must be a richer typology, taking into account the nature of the canon as well as the specific characteristics of the deuteronomic texts.
To sum up, then, I have argued that:
The main feature of Late Ancient and Medieval mathematical texts was their deuteronomic status.
This deuteronomic status accounts for the new types of practices present in those texts (often pejoratively referred to as “scholastic” or “pedantic”).
Taken together, these practices project a new image of mathematics as an ideal, unique, text-like object.
Ultimately, such images and practices change the nature of mathematics itself.
Thus, deuteronomic texts change mathematics. The very attempt to preserve a canon and work within it, inadvertently produced a new mathematics: conservatism worked as an instrument for change.
4.5 Acknowledgments
This article owes its inception to a presentation, followed by an especially vigorous discussion, in the QED conference at the Max Planck Institute for the History of Science, Berlin, May 1998. I wish to thank the convenor of the conference, Loraine Daston, and all of the discussants. I also wish to thank Karine Chemla, Catherine Goldstein and Alain Herreman for their inspiration in the preparation of this article.
4.6 References
Brashear, William (1994). Vier neue Texte zum antiken Bildungswesen. Archiv f ür Papyrusforschung 40:29–35.
Brentjes, Sonja (1998). Additions to Book I in the Arabic Traditions of Euclid’s Elements. Studies in History of Medicine and Science 15A(1–2):55–117.
Carroll, Lewis (1985). What the Tortoise Said to Achilles. Mind IV(14):278–80.
Chemla, Karine (1992). Résonances entre démonstration et procédure. Remarques sur le commentaire de Liu Hui (IIIe siècle) aux Neuf Chapitres sur les Procédures Mathématiques (Ier siècle). Extr ême-Orient Extrême-Occident 14:91–129.
Corry, Leo (1989). Linearity and Reflexivity in the Growth of Mathematical Knowledge. Science in Context 3(2):409–40.
Cuomo, Serafina (2000). Pappus of Alexandria and the Mathematics of Late Antiquity. Cambridge: Cambridge University Press.
Diadochus, Proclus (1992). Proclus: A Commentary on the First Book of Euclid’s Elements. Translated by Glenn R. Marrow. Princeton, NJ: Princeton University Press.
Euclid (888). Book X: Classification of incommensurables. In: Elements. MS D’Orville 301, The Bodleian Library.
Fowler, David H.F. (1987). The Mathematics of Plato’s Academy. Oxford: Clarendon Press.
Friedlein, Gottfried, ed. (1873). Procli Diadochi In Primum Euclids Elementorum Librum Commentarii. Leipzig: Teubner.
Goldstein, Catherine (1995). Un th éorème de Fermat et ses lecteurs. Saint-Denis: Presses Universitaires de Vincennes.
Heath, Thomas L. (1956). The Thirteen Books of Euclid’s Elements. 2nd ed. New York: Dover.
Heiberg, Johan Ludwig, ed. (1883). Euclids Elementa. Bibliotheca scriptorum Graecorum et Romanorum Teubneriana. Leipzig: Teubner.
– ed. (1895). Euclids Optica. Bibliotheca scriptorum Graecorum et Romanorum Teubneriana. Leipzig: Teubner.
– ed. (1910). Archimedes Opera. Vol. 1. Bibliotheca scriptorum Graecorum et Romanorum Teubneriana. Leipzig: Teubner.
– ed. (1913). Archimedes Opera. Vol. 3. Bibliotheca scriptorum Graecorum et Romanorum Teubneriana. Leipzig: Teubner.
Heiberg, Johan Ludwig and Rasmus O. Besthorn, eds. (1900). Codex Leidensis 399, I. Vol. II. Copenhagen: Hauniae Libraria Gyldendaliana Halle, Saale Universitäts- und Landesbibliothek.
Hogendijk, Jan P. (1985). Ibn al-Haytham’s Completion of the Conics. New York: Springer Verlag.
Høyrup, Jens (1990). Algebra and Naive Geometry: An Investigation of Some Basic Aspects of Old Babylonian Mathematical Thought. Altorientalische Forschungen 17: 27–69, 262–354.
Ibn al-Nadīm, Muḥammad ibn Isḥāq (1970). The Fihrist of al-Nadim; A Tenth-Century Survey of Muslim Culture. Ed. by Bayard Dodge. Translated by Bayard Dodge. Columbia University Press.
Jones, Alexander (1986). Book 7 of the Collection / Pappus of Alexandria. New York: Springer Verlag.
– (1994). Peripatetic and Euclidean Theories of the Visual Ray. Physis 31(1):47–76.
Knorr, Wilbur Richard (1989a). Textual Studies in Ancient and Medieval Geometry. Boston: Birkhäuser Boston.
– [1986] (1989b). The Ancient Tradition of Geometric Problems. Boston: Birkhäuser Boston.
– (1994). Pseudo-Euclidean Reflections in Ancient Optics: A Re-examination of Textual Issues Pertaining to the Euclidean Optica and Catoptrica. Physis 31(1):1–46.
– (1996). The Wrong Text of Euclid: On Heiberg’s Text and its Alternatives. Centaurus 38(2-3):208–76.
Mau, Jürgen and W. Mueller (1962). Mathematische Ostraka aus der Berliner Sammlung. Archiv f ür Papyrusforschung 17:1–10.
Mueller, Ian (1981). Philosophy of Mathematics and Deductive Structure in Euclid’s Elements. Cambridge, MA: MIT Press.
Netz, Reviel (1999a). Archimedes Transformed: The Case of a Result Stating a Maximum for a Cubic Equation. Archive for the History of Exact Sciences 54(1):1–48.
– (1999b). Proclus’ Division of the Mathematical Proposition into Parts: How and Why Was it Formulated? The Classical Quarterly 49(1):282–303.
– (1999c). The Shaping of Deduction in Greek Mathematics: A Study in Cognitive History. Cambridge: Cambridge University Press.
Saito, Ken (1997). Index of the Propositions Used in Book 7 of Pappus’ Collection. Jinbun Kenkyu: The Journal of Humanities (Faculty of Letters, Chiba University) 26(3):155–88.
Toomer, Gerald J., ed. (1984). Ptolemy’s Almagest. London: Duckworth.
– ed. (1990). Apollonius: Conics Books V to VII: The Arabic Translation of the Lost Greek Original in the Version of the Banū Mūsā. Sources in the History of Mathematics and Physical Sciences. New York: Springer Verlag.
Turner, Eric G., David H.F. Fowler, Ludwig Koenen, and Louise C. Youtie (1985). Euclid, Elements I, Definitions 1–10 (P. Mich iii 143). Yale Classical Studies 28:13–24.
Unguru, Sabetai (1975). On the Need to Rewrite the History of Greek Mathematics. Archive for the History of Exact Sciences 15:67–114.
– (1979). History of Ancient Mathematics: Some Reflections on the State of the Art. Isis 70(4):555–64.
Footnotes
Active in 4th century AD Alexandria, his biography is practically unknown. Dealing with a wide range of topics from arithmetic to mechanics, his most significant work is The Collection, a sort of mathematical encyclopaedia in eight books, nearly seven of which are extant. See Jones (1986); Cuomo (2000).
One can add at least one case of translation inside Greek culture itself, namely the translation of some works by Archimedes (Sphere and Cylinder, Method), from the original Doric dialect, into the dominant koine dialect.
See e.g. Knorr (1989a, 238–239, 812–816).
See especially Goldstein (1995) on the historical variability of such seemingly neutral concepts as “the obvious.”
Heiberg (1910, 162.25–164.11). It should be clear that much modern editorial work – and much subjective judgement – is implicit in any reference to “Archimedes” or to “the scholiast.” Still, this is a case where the two terms seem warranted, on linguistic and other grounds.
See Knorr (1996, 222–242), for a full discussion of this phenomenon of such, usually very brief, explications to arguments (e.g. in the form of cross-reference–on which more below).
Eutocius is the only commentator on Archimedes extant from antiquity. He was active in the sixth century AD. The diagram is taken from Netz (1999a, 19), where I discuss in detail the historical background to this text [Heiberg (1913, 140–146)], as well as develop the argument for showing the presence of “horizontal pedantry” there.
While case-analysis is by far the most common form of horizontal pedantry, other forms exist, e.g. explicitly proving converses left out by the original text (as Proclus does in his comment on Euclid’s Elements I.5), or that of providing a synthesis automatically recoverable from the original analysis (as Eutocius does with his alternative proof for Archimedes’ Sphere and Cylinder II.8 [Heiberg (1913, 206–12)]), or, finally, offering a complete analysis and synthesis pair when the original follows a direct synthetic approach (this is the case of the alternative proofs of Euclid’s Elements XIII.1–5, extant in some traditions).
I translate them by “enunciation,” “setting-out,” “definition of goal,” “construction,” “proof,” “conclusion,” but the reader should note there are other traditional translations for these terms. For a discussion, see Mueller (1981, 11–14).
Proclus was a 5th-century AD philosopher, among whose many extant works is a commentary on the first book of Euclid’s Elements: more philosophical than mathematical in its interests. The quotation is taken from that commentary [Friedlein (1873, 203–5)]. I use Morrow’s (1992) translation, substituting my terms for the parts (p. 159).
A detailed philological argument in favor of the later invention of this system by commentators is given in Netz (1999b).
The deep reason why such a gap between analysis and text occurred at all is that Proclus’ analysis is geared towards theorems (the more common type of proposition in Greek mathematics), while this first proposition happens to be a problem. Problems, in Euclid and elsewhere, tend not to have conclusions.
This is very common in Arabic translations: see e.g. Toomer (1990, passim).
This happens in some Latin traditions: see e.g. Knorr (1989a, 681–63).
The locus classicus for this is Pappus, Book III; see the discussions in Knorr ([1986] 1989b, 341–ff), as well as Cuomo (2000, Chap–4)–which is much more sensitive to the historical setting from which such schemes derive (in particular, Cuomo discusses in detail Pappus’ criticism of the purported solution I mention).
On the structure of this work by Euclid, see Mueller (1981, 41–52).
Talk given at the Fourth International Conference on Greek Mathematics, Les Treilles, July 1998.
See Knorr (1989a, part–III) for the argument that the extant text is an abridgement–and for an ambitious attempt at following the historical process of this abridgement.
Friedlein (1873, 361–22). We know nothing about the date of Aigeias.
The enunciations are (following Heath’s (1956) translation): (27) “If a straight line falling on two straight lines make the alternate angles equal to one another, the straight lines will be parallel to one another,” (28) “If a straight line falling on two straight lines make the exterior angle equal to the interior and opposite angle on the same side, or the interior angles on the same side equal to two right angles, the straight lines will be parallel to one another.” A possible Aigeias version: “If a straight line falling on two straight lines make the alternate angles equal to one another, or it make the exterior angle equal to the interior and opposite angle on the same side, or it make the interior angles on the same side equal to two right angles, the straight lines will be parallel to one another.”
I ignore a few papyri which reveal an acquaintance with the contents of Euclid, without being “texts of Euclid,” such as the important series of ostraka from the third century BC (discussed in Mau and Mueller (1962)).
For this and for the next papyrus, see Fowler (1987, 209–ff).
Notice that an important advantage of such an epitome is that it could obviously “squeeze” all of Euclid inside a single papyrus roll.
Friedlein (1873, 232.11–12, 251.2, 260.10, 426.11).
Jones’ position is that clearly both versions are not Euclid’s “original text,” although he suspects version B is closer in its mathematical content to the original (personal communication). Interestingly, Jones (1994) mentions the more standard lettering of version A as an argument in favor of its being, in my own terminology, “deuteronomic.” In his view, an original deviant system of lettering has indeed been ’corrected’ in some later edition. This is possible, too: an example of the fact that the dynamics of deuteronomic texts can sometimes lead in opposite directions (cf. comments above on “epitome”). For my immediate purpose, however, it is sufficient to take note of Jones’ agreement to the view according to which version B may indeed be a deuteronomic text.
This is of course an acronym: K for kentron, “center.”
I adapt, of course, Peirce’s notion of “symbol,” as a sign signifying by convention: see the discussion of the application of this terminology to Greek mathematics, in Netz (1999c, 47).
While such a discussion goes beyond the scope of this article, I will argue that ancient sources, such as Plato and Aristotle, think of mathematics predominantly in the context of individual acts of proof, not in the context of a single, idealised Mathematics. I hope to pursue this argument in a separate article. Note however that since this image of mathematics is so well-known, I hardly find it necessary to document in detail its presence in late antiquity and the Middle Ages: I refer in the text to two very typical examples, from Greek late antiquity and the Islamic world, respectively (besides, of course, referring to the totality of the practices documented in the first section of this article.)
Beginning already in late antiquity: see Friedlein (1873, 191–195, 362–375).